Eric Chong
LAB 18: A LAB PROBLEM--MOMENT OF INERTIA and FRICTIONAL TORQUE
Lab Partners: Nina Song and Joel Cook
Purpose: The goal of this lab is to measure the time it takes for a cart tied to a string connected to a large metal disk on a central shaft, by measuring and calculating the total moment of inertia and the acceleration the cart will experience.
Theory/Introduction: The moment of inertia is different for each individual objects. However, the moment of inertia of a system can be calculated through the sum of the individual moments of inertia of the individual objects. In the net torque equation, the net torque is equal to inertia times the angular acceleration. We can find the angular acceleration through video analysis. With this, it is possible to calculate the net torque the system has, and by using the relationship with the net force equation, it is possible to solve for the acceleration of the cart.
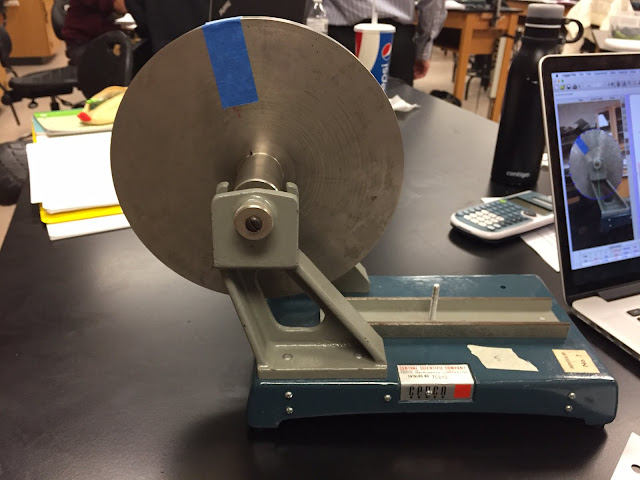
Procedure: We first start this lab by measuring the radius and height of each cylinder (2 small and 1 large) of the apparatus that looks like this:
After measuring the radius and height of each cylinder, we started calculating the volume of each and totaling the volume. We do this because the cylinders have uniform mass, meaning that the volume is proportional to the mass. If we can get the percent volume of each individual cylinder, we can figure out their individual masses, given the total mass of the apparatus. And once we have their masses, we can calculate for their individual moments of inertia. And finally, we can get the total moment of inertia by adding each of their moments of inertia together. Here are the measurements and calculations we did:
(To make calculations easier, we assumed that the two smaller cylinders have the same radii and height.)
Our total moment of inertia came out to be 0.0199 kg*m^2, which is what we expected based on what the professor said. Next, we started doing video analysis by recording the spin of the apparatus, in order to measure its angular acceleration. We used LoggerPro for this part and plotted points on the rim of the big cylinder as it was spinning. Through this, we can analyze the angular acceleration by looking at the slope of the angular velocity vs. time graph. We derived the angular velocity equation in LoggerPro by inputting the equation, omega = (Vtangential)/R, where omega is the angular velocity. Here is what the graphs looks like:
(This is the position vs. time graph)
(Here is the omega vs. time graph)
The angular acceleration, by looking at the slope, is -0.1903 rad/s^2. We also quickly measured the angle of the slope the cart is traveling on, which turns out to be 40 degrees. With this, we now have all of the variables needed to calculate the acceleration of the cart. We set up two equations. One is the net force equation, and the other is the net torque equation. Through manipulation, we are able to solve for the acceleration the cart experiences, and in turn the time it takes for the cart to travel 1 meter, the length of the slope. Here is the calculation:
Our calculation shows that the time it takes is approximately 7.0515 seconds. We then ran 5 trials to test the calculation. Here is what we got:
It is seen that all of the trials are above the calculated value, which is a sign for a sort of systematic error. Perhaps the pulley is not as ideal as we thought, and may have contributed a slight frictional torque on the system. Or perhaps the slope that the cart was traversing on is not entirely frictionless. Or maybe that the way the string is strung on the cylinder is a contributing factor to the slight error in the measurements, in that maybe the tension is not quite equal to mgsin(theta), because as the cart was going down, the tension might be changing as the cart is speeding up.
Conclusion: The lab is successful, in that even though our data is not quite as what we expected, we were able to maintain an error within 5%. This still confirms that our method is still largely correct, and that perhaps we need some slight adjustments with the pulley, the slope, or the cart in order to provide better data closer to the calculations. Some sources of uncertainty may have come from the pulley itself, since frictional torque is a possibility, the slope itself, since it could have had friction on the cart, or the string, since I suspect that there might be a sort of changing tension as the cart was speeding up. Overall, the lab showcases how to calculate the moments of inertia of each individual piece, and adding them up in order to find the total moment of inertia, how to find the angular acceleration through video analysis, and how both the net force and net torque equations are related and can be used to find the acceleration of the cart.





No comments:
Post a Comment