Eric Chong
Lab 17: PHYSICS 4A LAB--Finding the moment of inertia of a uniform triangle.
Lab Partners: Nina Song and Joel Cook
Purpose: To determine the moment of inertia of a right triangular thin plate around its center of mass, for two perpendicular orientations of the triangle.
Theory/Introduction: For this lab, we will be exercising the concept of the summation of moments of inertia in order to calculate the moment of inertia of the entire system. This time, we will be using the reverse, by taking the moment of inertia of the system in order to find the moment of inertia of an individual triangle plate. We will be using this theory and another approach, the approach of using the parallel axis theorem to calculate the moment of inertia of the triangle at its center of mass, and comparing the two in order to validate our results. By doing this, we can confirm that the two concepts are equally valid for calculating the moment of inertia of the triangle.
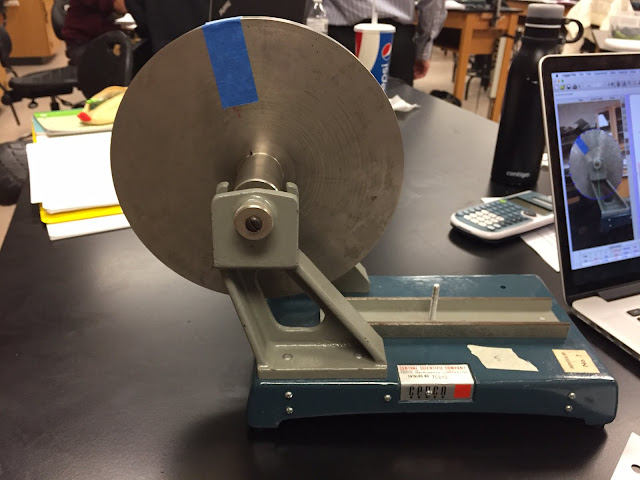
Procedure: We start by using the same setup as the Rotational acceleration lab. Here is what the apparatus looks like:
We use this apparatus and LoggerPro in order to measure the angular acceleration of the apparatus. This is so we can use the equation that we developed in a previous lab. The equation will be shown in the calculation. We first measured the angular acceleration without the triangle. Before we launched the apparatus, we measured the mass of the hanging mass, which turns out to be 25 grams or 0.025 kg, and the radius of the pulley, which turns out to be 0.0248 m. We did this for the calculations portion. We then launched the apparatus and measured its angular acceleration by looking at the slope of the velocity vs. time graph. Here is what the graph looks like:
Here we can see that the angular accelerations going up and down are different. We combat this by taking the average of the two. Next, we calculated the moment of inertia of the apparatus by doing this calculation:
(Note: m is the hanging mass, r is the radius of the pulley, and the denominator is the average of the angular acceleration going up and down.)
We found the moment of inertia of the apparatus alone to be 0.000965387 kg*m^2. With this we can then calculate the moment of inertia of the triangle by doing the same procedure we did, but instead placing the triangle on the apparatus in an upright orientation, calculating the moment of inertia with the same equation, and then subtracting the result with the moment of inertia of the apparatus alone. Here is the apparatus with the upright triangle looks like:
Here is what the graph on LoggerPro looks like after we launched the apparatus:
After finding the angular accelerations and taking the average, we then performed the same calculation for this apparatus-triangle system. Here is the calculation:
The last part of the calculation is simply taking the difference between the moment of inertia of the apparatus-triangle system, which is 0.001239333 kg*m^2, with the moment of inertia of the apparatus alone. The moment of inertia of the upright triangle turns out to be 0.000273946 kg*m^2.
For the next part, we turned the triangle sideways, so that its longer side becomes the base. Here is what it looks like:
We performed the same procedure as before, so here is the graph with the angular acceleration:
Here is the calculation:
The moment of inertia of the sideways triangle turns out to be 0.000588837 kg*m^2.
We now want to confirm our calculations by using the other approach, the approach using the parallel axis theorem in order to find the center of mass moment of inertia of the triangles. The results should be the same or close. Here is the derivation for the moment of inertia of the triangles at the center of mass:
We found that the moment of inertia at the triangle's center of mass is (1/18)MB^2, with M being the mass of the triangle, and B being the base of the triangle. With this, we measured the mass of the triangle, which turns out to be 0.456 kg. For the shorter base of the triangle, we found that the length is 98.2 mm or 0.0982 m. For the longer base of the triangle, we found that the length is 149.1 mm or 0.1491 m. With these measurements, we can finally perform the calculations. Here are the calculations:
Looking at these calculations, it seems that the two types of approaches validate each other. This means that both ways are valid ways of finding the moment of inertia of the triangle.
Conclusion: The lab is a success in that we were able to find the moment of inertia of the upright and sideways triangle using two different approaches. One way is the use of the angular acceleration and measuring the mass of the hanging mass and the radius of the pulley and using the desired equation that we used in a previous lab. The other way is to use the moment of inertia of the triangle at its center of mass, and plugging in the mass of the triangle and their respective bases. Both approaches confirm that the moments of inertia of the upright and sideways triangle are close to the calculations. Some sources of uncertainty could come from our measurements of mass, length, base, radius, etc. Another source of uncertainty could come from the pulley, as it is not an ideal pulley, as seen in the differing angular accelerations going up and down. Overall, this lab is successful and we were able to learn that both ways of calculating the moment of inertia of the triangles are valid.